Quantum Energy Lines and the optimal output ergotropy problem

2021-11-24 News, Seminars

Join us online on Wednesday, November 24th at 14:00 CET (13:00 GMT) for an ICTQT seminar

Rescaled maximum output ergotropy values E(max)E,G/E attainable with Gaussian inputs with input energy E for one-mode, not PI BGCs. (a),(b) Attenuator-squeezing channels Γη,ζ=Lη,0∘Σζ with η=0.5 and η=0.9, respectively. (c),(d) Amplifier-sqeezing channels Θμ,ζ=Aμ,0∘Σζ with μ=2 and μ=5, respectively. In the no-squeezing ζ=1 regime (blue lines) the maps are PI, and the reported values coincide with the absolute maxima (η and μ) dictated by Theorem 2.

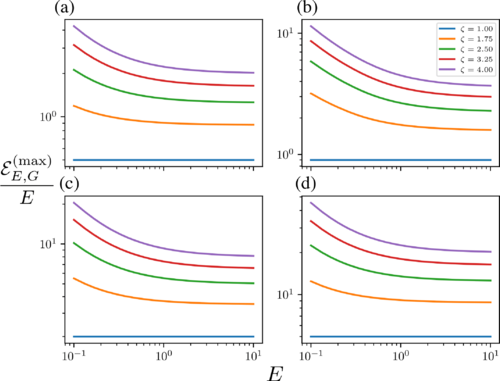
We study the possibility of conveying useful energy (work) along a transmission line that allows for a partial preservation of quantum coherence. As a figure of merit we adopt the maximum values that ergotropy, total ergotropy, and non-equilibrium free-energy attain at the output of the line for an assigned input energy threshold. When the system can be modelled in terms of Phase-Invariant Bosonic Gaussian Channels (BGCs), we show that coherent inputs are optimal. For generic BGCs which are not Phase-Invariant the problem becomes more complex and coherent inputs are no longer optimal. In this case, focusing on one-mode channels, we solve the optimization problem under the extra restriction of Gaussian input signals.

This talk is based on https://journals.aps.org/prl/abstract/10.1103/PhysRevLett.127.210601.

Tags:

You may also like

Skip to content